A wallpaper group is a mathematical classification of a two-dimensional repetitive pattern, based on the symmetries in the pattern. There is 17 wallpaper groups. I made an example of each one with a domain coloring technique. I'd like to thank Frank A. Farris for his amazing book "Creating Symmetry".

I'll first show the 17 groups, then how we can play with them by morphing them or animating them.

The 17 groups

P1

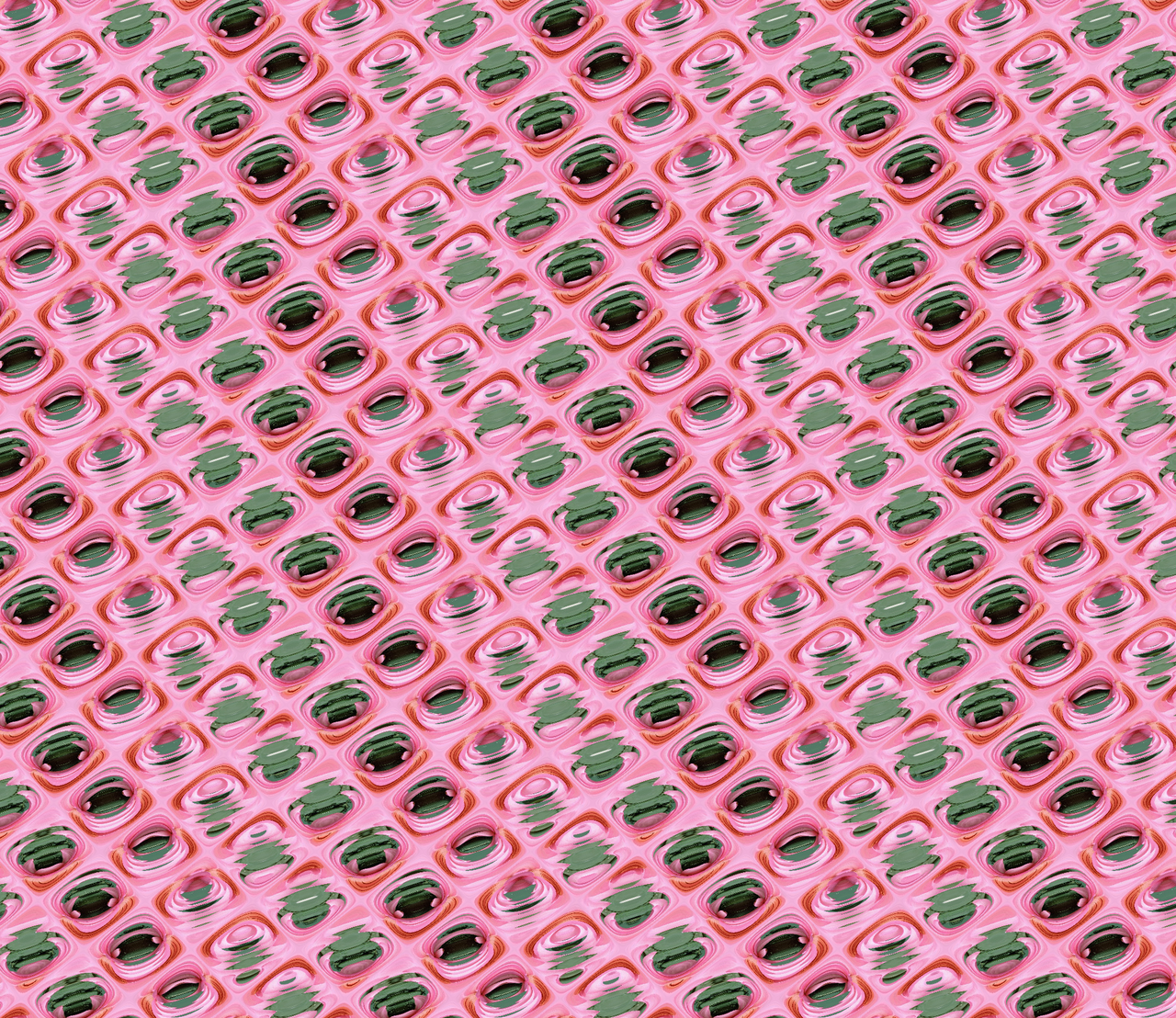
Pm

Pg

Pmm

Pmg

Pgg

Cm

Cmm

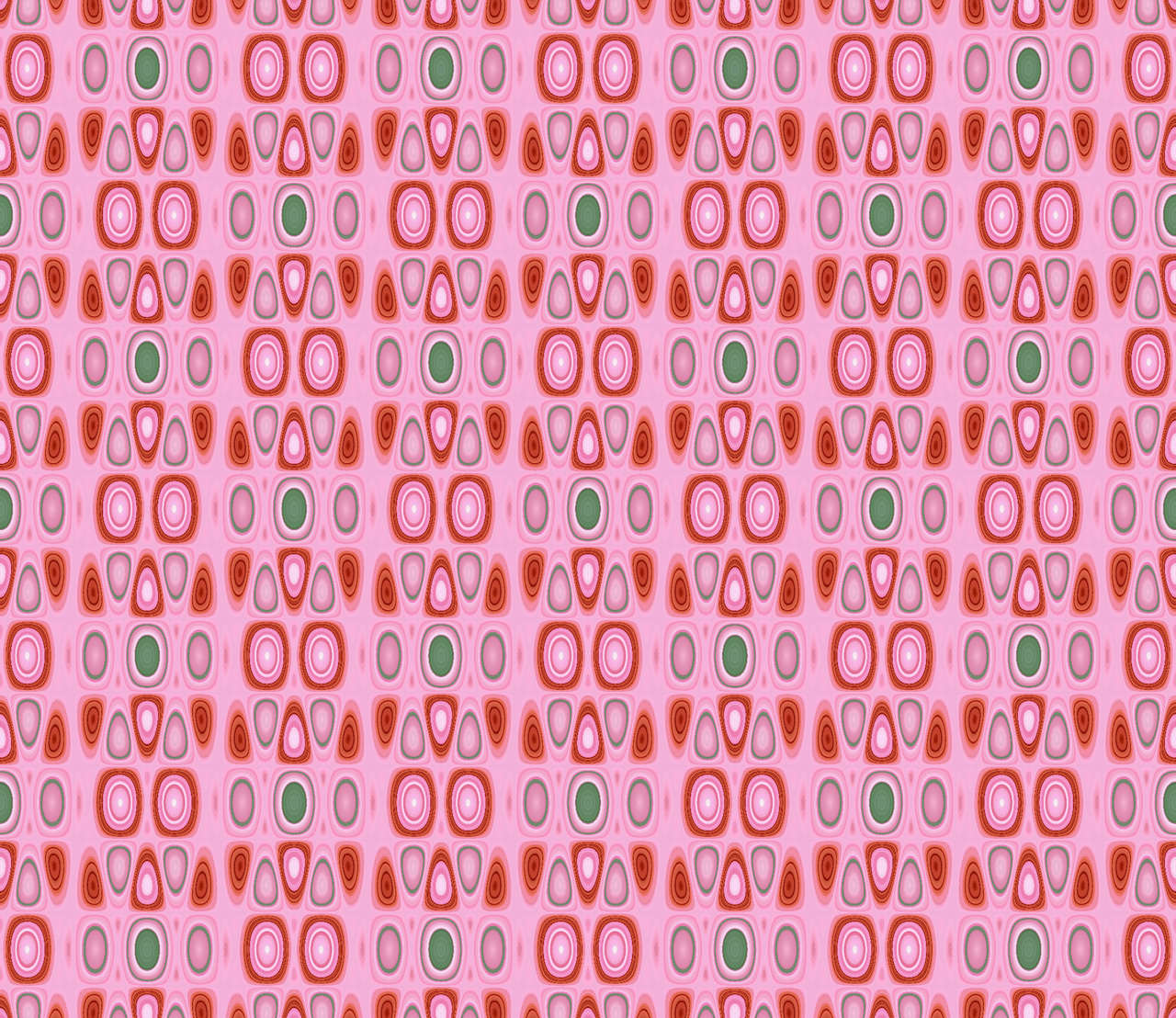
P2

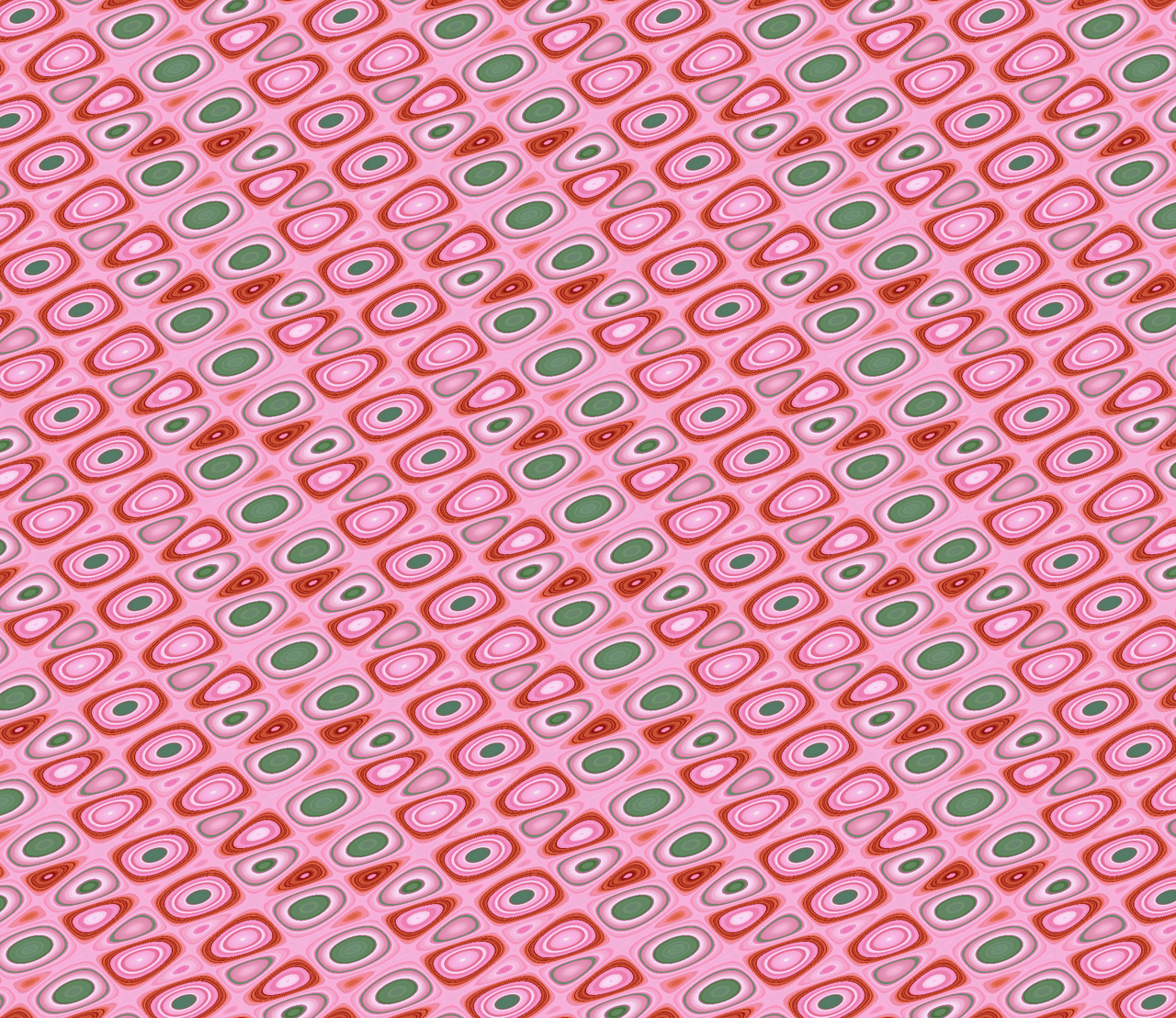
P3

P31m

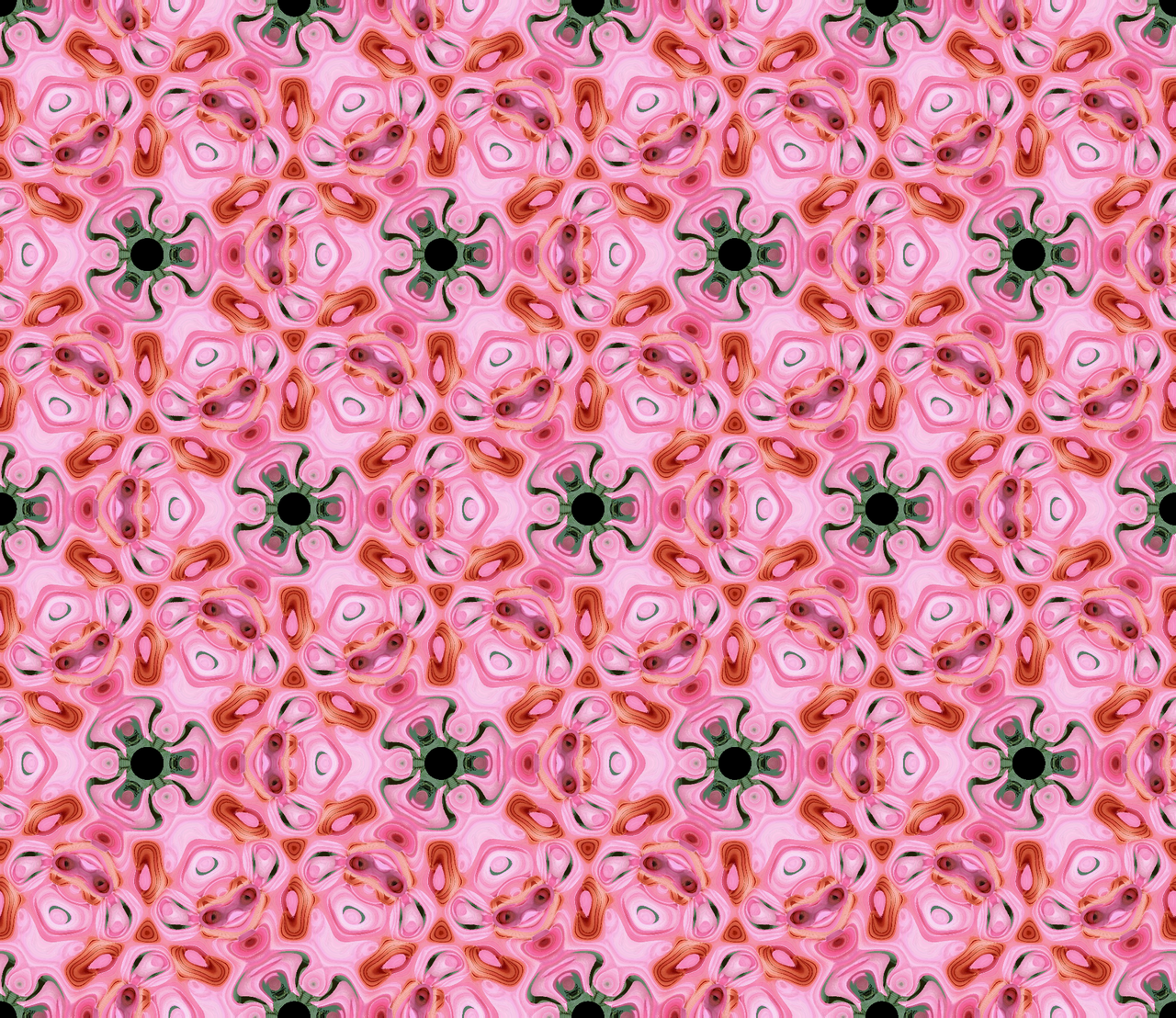
P3m1

P4

P4m

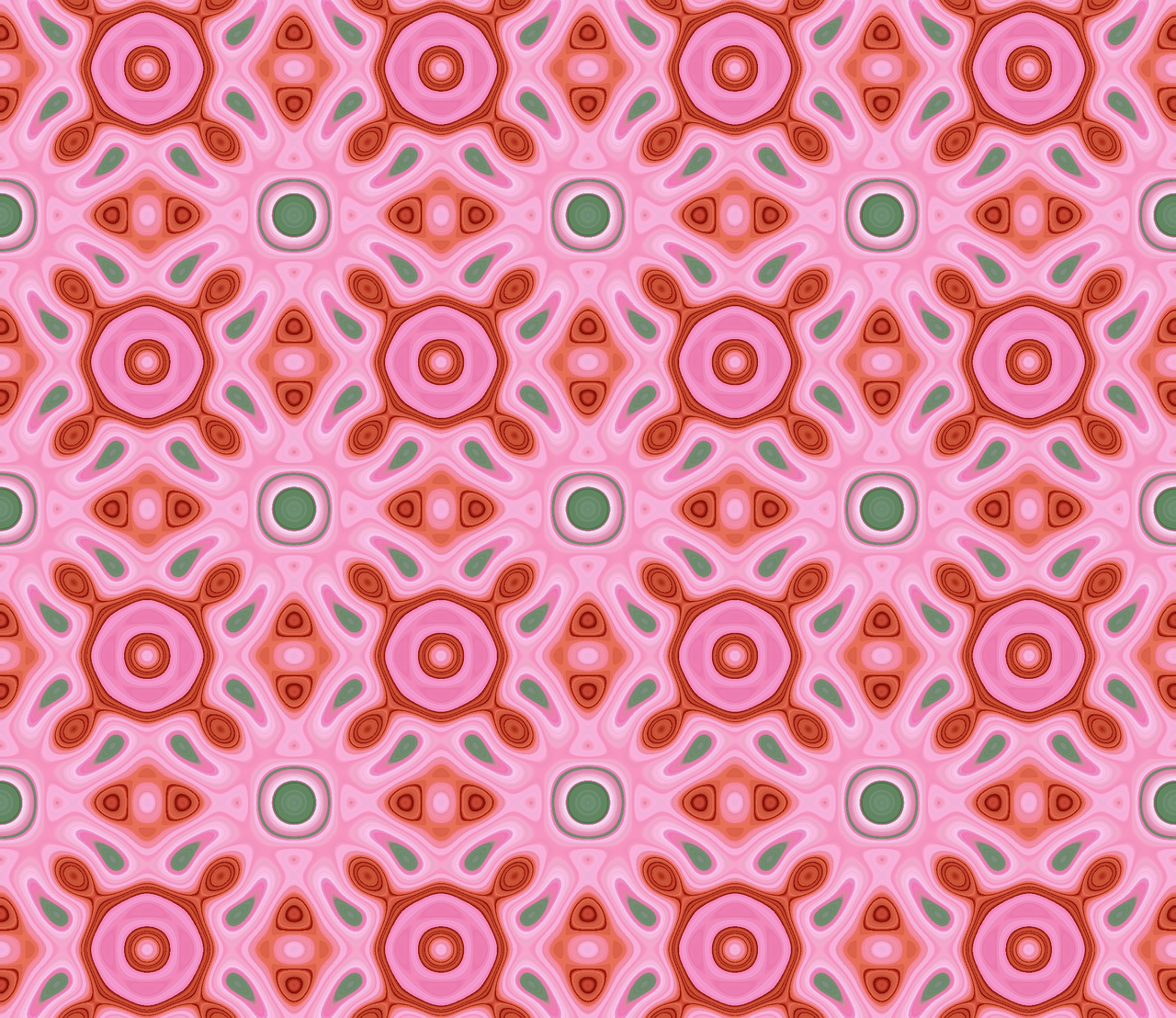
P4g

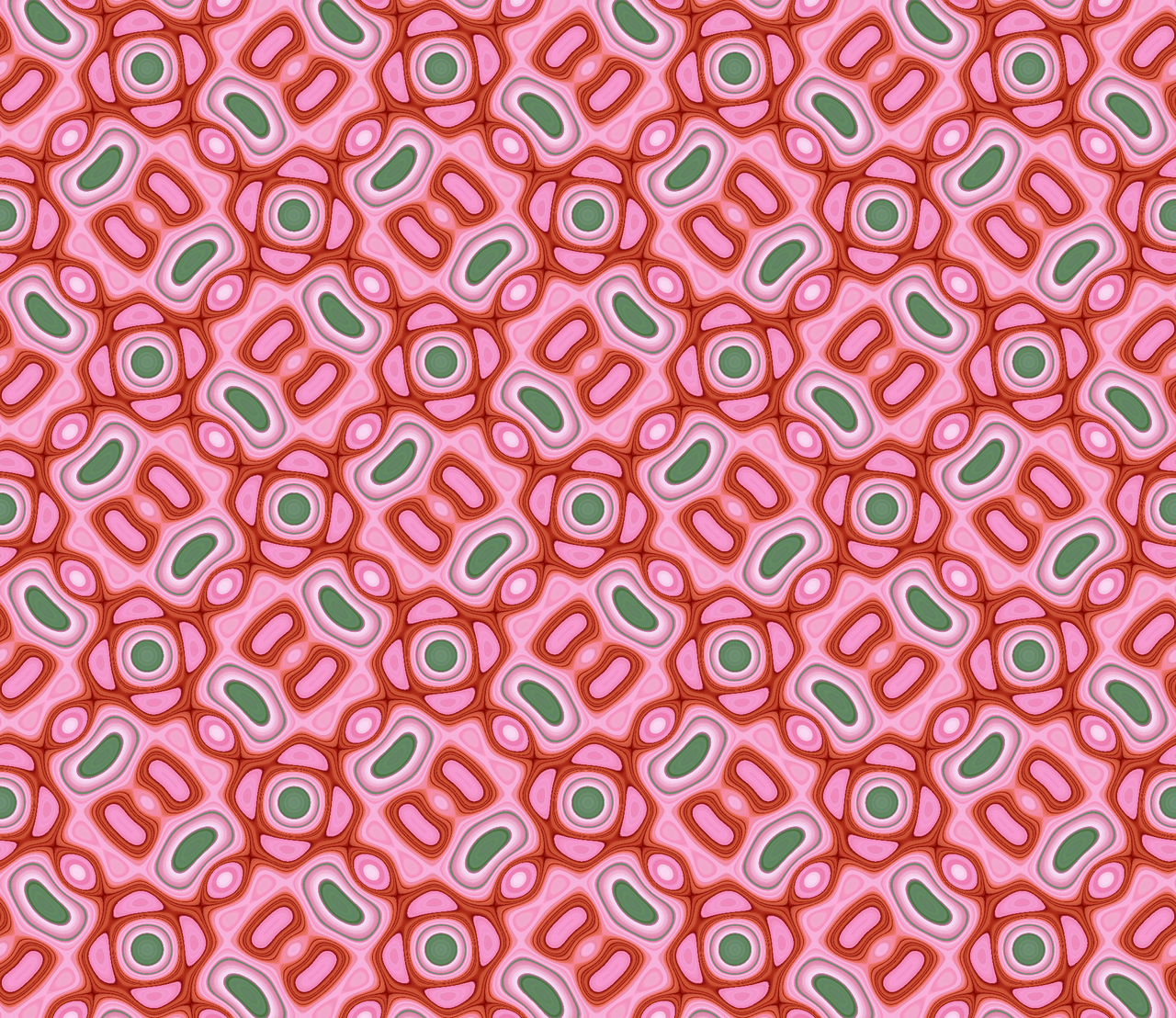
P6

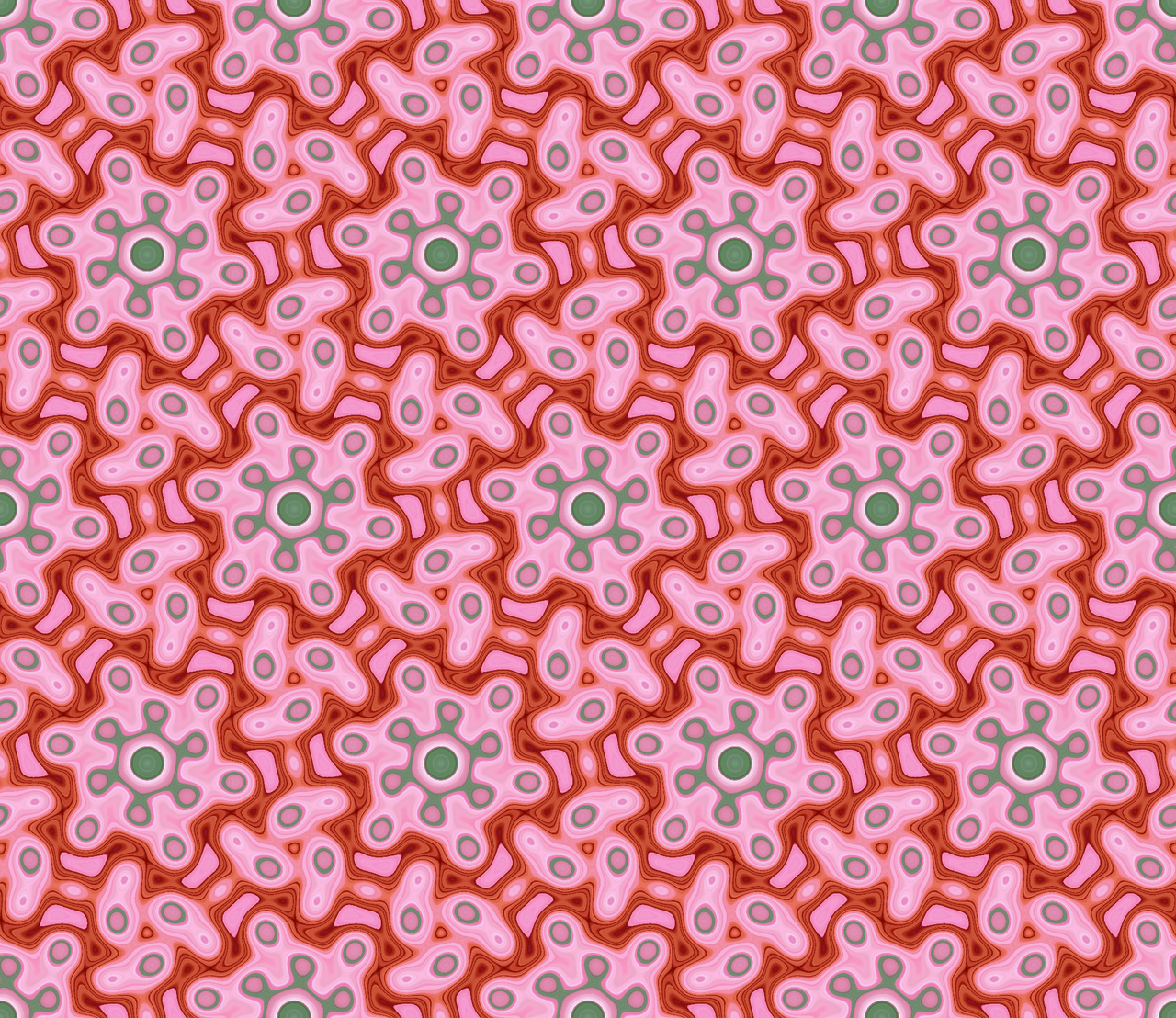
P6m

Morphing wallpapers

Animated wallpapers